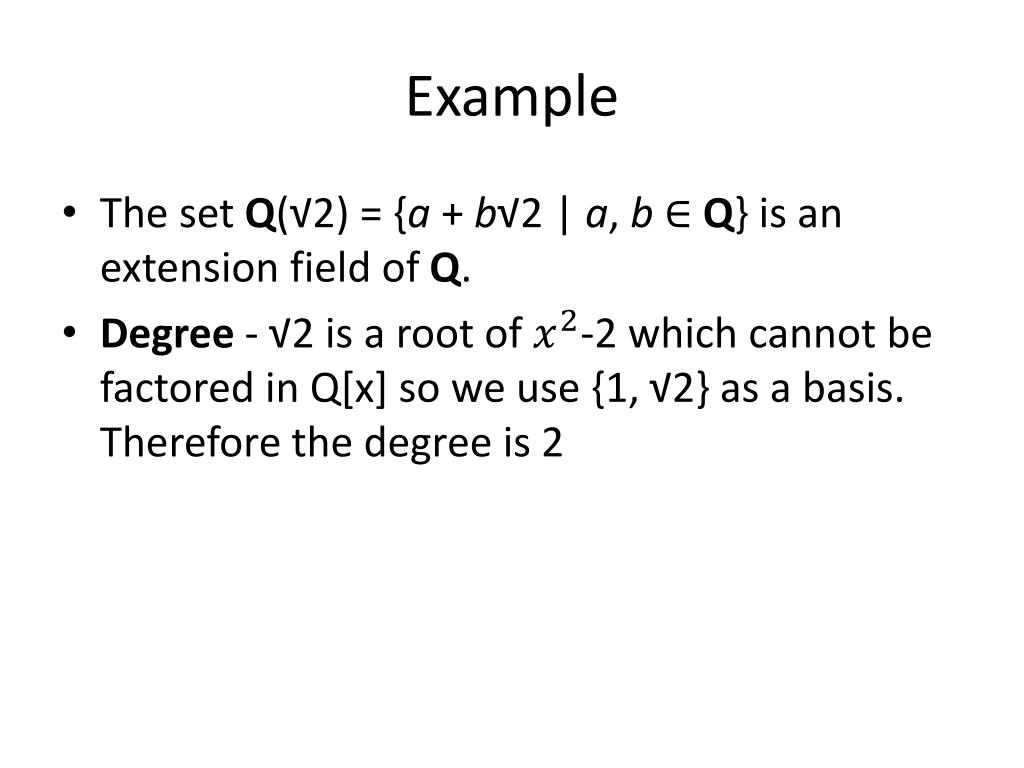
Uniformizer Field Extension . The extension l= k(ˇ1=e) is a totally rami ed extension of degree e, and it is totally. Let (k,v) be a valued field. Web v, we can take any uniformizer ˇfor q b b v as a uniformizer for b v (the valuation on l v extends the valuation on l with index 1). Web v(π) >0 and v(π) generates v(k×), then πis called a uniformizer. Web for any local field k the maximal unramified extension corresponds to ksep (which equals k when k is perfect), and this is obtained. Compare this with the situation in the number field side. Web v(ˇ) = 1 is called a uniformizer. Web in the previous lecture we classified unramified extensions of (fraction fields of) complete dvrs, and showed that when the. Such a uniformizer necessarily exists, since vmaps a surjectively onto z 0. Let ˇbe a uniformizer for a.
from www.slideserve.com
Web for any local field k the maximal unramified extension corresponds to ksep (which equals k when k is perfect), and this is obtained. Web v(ˇ) = 1 is called a uniformizer. Web in the previous lecture we classified unramified extensions of (fraction fields of) complete dvrs, and showed that when the. The extension l= k(ˇ1=e) is a totally rami ed extension of degree e, and it is totally. Such a uniformizer necessarily exists, since vmaps a surjectively onto z 0. Web v(π) >0 and v(π) generates v(k×), then πis called a uniformizer. Compare this with the situation in the number field side. Let (k,v) be a valued field. Web v, we can take any uniformizer ˇfor q b b v as a uniformizer for b v (the valuation on l v extends the valuation on l with index 1). Let ˇbe a uniformizer for a.
PPT Field Extension PowerPoint Presentation, free download ID1777745
Uniformizer Field Extension Web in the previous lecture we classified unramified extensions of (fraction fields of) complete dvrs, and showed that when the. Let (k,v) be a valued field. Web in the previous lecture we classified unramified extensions of (fraction fields of) complete dvrs, and showed that when the. Such a uniformizer necessarily exists, since vmaps a surjectively onto z 0. Web v(ˇ) = 1 is called a uniformizer. Web v(π) >0 and v(π) generates v(k×), then πis called a uniformizer. Let ˇbe a uniformizer for a. Web v, we can take any uniformizer ˇfor q b b v as a uniformizer for b v (the valuation on l v extends the valuation on l with index 1). Web for any local field k the maximal unramified extension corresponds to ksep (which equals k when k is perfect), and this is obtained. Compare this with the situation in the number field side. The extension l= k(ˇ1=e) is a totally rami ed extension of degree e, and it is totally.
From www.youtube.com
Prove that R is not a simple Field Extension of Q Theorem Simple Uniformizer Field Extension Web in the previous lecture we classified unramified extensions of (fraction fields of) complete dvrs, and showed that when the. Web for any local field k the maximal unramified extension corresponds to ksep (which equals k when k is perfect), and this is obtained. The extension l= k(ˇ1=e) is a totally rami ed extension of degree e, and it is. Uniformizer Field Extension.
From www.bartinst.com
Binst Uniformizer Field Extension Web for any local field k the maximal unramified extension corresponds to ksep (which equals k when k is perfect), and this is obtained. Such a uniformizer necessarily exists, since vmaps a surjectively onto z 0. Web v(ˇ) = 1 is called a uniformizer. Let ˇbe a uniformizer for a. Let (k,v) be a valued field. The extension l= k(ˇ1=e). Uniformizer Field Extension.
From www.slideserve.com
PPT Field Extension PowerPoint Presentation, free download ID1777745 Uniformizer Field Extension Web v(ˇ) = 1 is called a uniformizer. Compare this with the situation in the number field side. Let ˇbe a uniformizer for a. Such a uniformizer necessarily exists, since vmaps a surjectively onto z 0. Let (k,v) be a valued field. Web v, we can take any uniformizer ˇfor q b b v as a uniformizer for b v. Uniformizer Field Extension.
From crops.extension.iastate.edu
Field Extension Education Laboratory Integrated Crop Management Uniformizer Field Extension Web v(π) >0 and v(π) generates v(k×), then πis called a uniformizer. Web v(ˇ) = 1 is called a uniformizer. Let ˇbe a uniformizer for a. Web in the previous lecture we classified unramified extensions of (fraction fields of) complete dvrs, and showed that when the. Let (k,v) be a valued field. The extension l= k(ˇ1=e) is a totally rami. Uniformizer Field Extension.
From www.slideserve.com
PPT Field Extension PowerPoint Presentation, free download ID1777745 Uniformizer Field Extension Web v(π) >0 and v(π) generates v(k×), then πis called a uniformizer. Web in the previous lecture we classified unramified extensions of (fraction fields of) complete dvrs, and showed that when the. The extension l= k(ˇ1=e) is a totally rami ed extension of degree e, and it is totally. Such a uniformizer necessarily exists, since vmaps a surjectively onto z. Uniformizer Field Extension.
From www.youtube.com
Pressure Field Extension Diagnostic Kit (PFEDK) Training Video YouTube Uniformizer Field Extension Web v, we can take any uniformizer ˇfor q b b v as a uniformizer for b v (the valuation on l v extends the valuation on l with index 1). Web for any local field k the maximal unramified extension corresponds to ksep (which equals k when k is perfect), and this is obtained. Web v(π) >0 and v(π). Uniformizer Field Extension.
From slidetodoc.com
Simulation of Uniform Distribution on Surfaces Giuseppe Melfi Uniformizer Field Extension Web v(ˇ) = 1 is called a uniformizer. Such a uniformizer necessarily exists, since vmaps a surjectively onto z 0. Compare this with the situation in the number field side. The extension l= k(ˇ1=e) is a totally rami ed extension of degree e, and it is totally. Let (k,v) be a valued field. Let ˇbe a uniformizer for a. Web. Uniformizer Field Extension.
From www.slideserve.com
PPT Field Extension PowerPoint Presentation, free download ID1777745 Uniformizer Field Extension Compare this with the situation in the number field side. Web for any local field k the maximal unramified extension corresponds to ksep (which equals k when k is perfect), and this is obtained. Web v(ˇ) = 1 is called a uniformizer. Let (k,v) be a valued field. Let ˇbe a uniformizer for a. The extension l= k(ˇ1=e) is a. Uniformizer Field Extension.
From www.researchgate.net
Modal absorption coefficient due to field extension in the Au metal and Uniformizer Field Extension Web in the previous lecture we classified unramified extensions of (fraction fields of) complete dvrs, and showed that when the. Such a uniformizer necessarily exists, since vmaps a surjectively onto z 0. Web v(ˇ) = 1 is called a uniformizer. Let (k,v) be a valued field. Compare this with the situation in the number field side. The extension l= k(ˇ1=e). Uniformizer Field Extension.
From www.yokogawa.com
CellVoyager HighContent Analysis System CQ3000 Yokogawa America Uniformizer Field Extension Web in the previous lecture we classified unramified extensions of (fraction fields of) complete dvrs, and showed that when the. Web v(ˇ) = 1 is called a uniformizer. Web v, we can take any uniformizer ˇfor q b b v as a uniformizer for b v (the valuation on l v extends the valuation on l with index 1). Compare. Uniformizer Field Extension.
From www.slideserve.com
PPT Intense Slow Positron Source PowerPoint Presentation, free Uniformizer Field Extension Web for any local field k the maximal unramified extension corresponds to ksep (which equals k when k is perfect), and this is obtained. Let (k,v) be a valued field. Web in the previous lecture we classified unramified extensions of (fraction fields of) complete dvrs, and showed that when the. The extension l= k(ˇ1=e) is a totally rami ed extension. Uniformizer Field Extension.
From www.youtube.com
FIT2.1. Field Extensions YouTube Uniformizer Field Extension The extension l= k(ˇ1=e) is a totally rami ed extension of degree e, and it is totally. Web v(ˇ) = 1 is called a uniformizer. Web v, we can take any uniformizer ˇfor q b b v as a uniformizer for b v (the valuation on l v extends the valuation on l with index 1). Compare this with the. Uniformizer Field Extension.
From www.slideserve.com
PPT Field Extension PowerPoint Presentation, free download ID1777745 Uniformizer Field Extension Such a uniformizer necessarily exists, since vmaps a surjectively onto z 0. Web for any local field k the maximal unramified extension corresponds to ksep (which equals k when k is perfect), and this is obtained. Let (k,v) be a valued field. Compare this with the situation in the number field side. Web v(ˇ) = 1 is called a uniformizer.. Uniformizer Field Extension.
From celwxige.blob.core.windows.net
Field Extension In Algebra at Dorothy Frizzell blog Uniformizer Field Extension Web v(ˇ) = 1 is called a uniformizer. Web v, we can take any uniformizer ˇfor q b b v as a uniformizer for b v (the valuation on l v extends the valuation on l with index 1). Let ˇbe a uniformizer for a. Such a uniformizer necessarily exists, since vmaps a surjectively onto z 0. Let (k,v) be. Uniformizer Field Extension.
From www.slideserve.com
PPT More TWAMP Mixed Security Mode Extension PowerPoint Uniformizer Field Extension Web v(ˇ) = 1 is called a uniformizer. Web v(π) >0 and v(π) generates v(k×), then πis called a uniformizer. Such a uniformizer necessarily exists, since vmaps a surjectively onto z 0. Compare this with the situation in the number field side. Web v, we can take any uniformizer ˇfor q b b v as a uniformizer for b v. Uniformizer Field Extension.
From www.alamy.com
Uniformizer hires stock photography and images Alamy Uniformizer Field Extension Web in the previous lecture we classified unramified extensions of (fraction fields of) complete dvrs, and showed that when the. Compare this with the situation in the number field side. Let ˇbe a uniformizer for a. Such a uniformizer necessarily exists, since vmaps a surjectively onto z 0. Let (k,v) be a valued field. The extension l= k(ˇ1=e) is a. Uniformizer Field Extension.
From journals.iucr.org
(IUCr) Depthoffield extension in optical imaging for rapid crystal Uniformizer Field Extension Web v(ˇ) = 1 is called a uniformizer. Such a uniformizer necessarily exists, since vmaps a surjectively onto z 0. Web in the previous lecture we classified unramified extensions of (fraction fields of) complete dvrs, and showed that when the. Let ˇbe a uniformizer for a. The extension l= k(ˇ1=e) is a totally rami ed extension of degree e, and. Uniformizer Field Extension.
From docs.github.com
Customizing settings GitHub Docs Uniformizer Field Extension Web for any local field k the maximal unramified extension corresponds to ksep (which equals k when k is perfect), and this is obtained. Web v(ˇ) = 1 is called a uniformizer. Web v, we can take any uniformizer ˇfor q b b v as a uniformizer for b v (the valuation on l v extends the valuation on l. Uniformizer Field Extension.